There is an erroneous view of fractional reserve banking that claims all fractionally reserved banks are de facto insolvent. A variant asserts that if a bank takes in $100 of deposits then it can make $1000 of loans, creating money out of thin air.
This view is false.
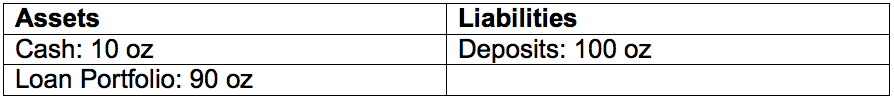
To understand why, let’s look at the bank’s balance sheet. The following examples are presented as being for one bank, but could as easily represent the consolidated balance sheet of the entire banking sector. Ignoring shareholder equity, and other complicating factors that would make this analysis harder to follow, the bank takes the $100 deposit:
Now assuming they could lend $1000, what would this look like?
This could never work. Assets have to equal liabilities, but if a bank creates loans out of thin air then it would be creating assets.
Fractional reserve lending is not lending more than what the bank takes in via deposits. That would be impossible. The bank lends less than it takes in deposits.
Aggregate deposits in the banking system (and thus bank debt) can (and typically do) exceed the amount of base money in the system. For the remainder of this article, we will look at the gold standard with fractional reserves, to make it simpler and clearer. First, someone deposits some gold coin into a bank.
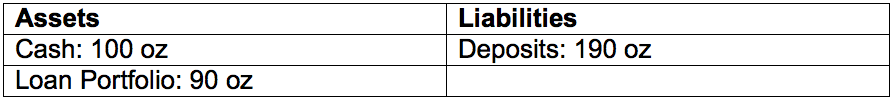
So far, so good. Next, the bank makes a loan of more than zero but less than the total deposited.
It’s still solvent. Now let’s say that the borrower pays the 90 oz to a contractor to build a new house and the contractor deposits the money in the same bank.
What just happened here? The size of the balance sheet increased. The balance sheet shows assets to match the liabilities; there is no evidence of insolvency yet. The bank may or may not be insolvent.
To drill down further, we need to introduce the idea of duration. Every deposit has a duration (for a demand deposit, duration is effectively zero), and every loan also has a duration.
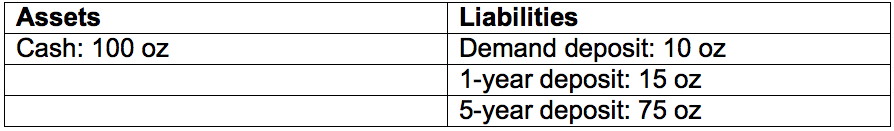
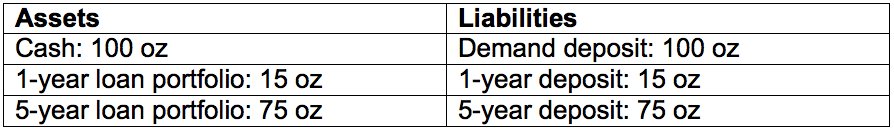
So let’s go back to the original depositor. He put in 100 oz of gold, asking the bank to keep 10 oz for withdrawal on demand, 15 oz to be withdrawn in one year, and 75 oz to be withdrawn in five years.
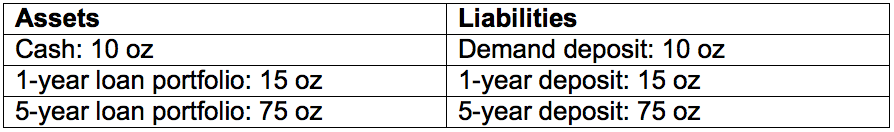
Now the bank makes two loans, a one-year loan of 15 oz and a five-year loan of 75 oz.
This is still a solid balance sheet. Now let’s say the borrowers of those loans pay people who deposit the 90 oz of gold back into the bank as demand deposits.
There is still no problem. The maturities of the bank’s assets match its liabilities. This bank is perfectly solvent. (In the real world, the bank would set aside its own capital as loan loss reserves to cover the credit risk).
Before proceeding to duration mismatch, which is a serious problem, let’s address the fact that the balance sheet has expanded. Some would argue that the bank has just expanded the “money supply”. This is not true; the same 100 oz of gold is still in the system. What has been expanded is credit.
One side of credit is the asset on the books. To the bank, the loans it extended are assets. These assets have real value based on the expectation to be repaid, and they can be sold to other banks, etc. The other side is the liability. To the bank, the deposits it accepted are liabilities.
The bank has increased both its assets and its liabilities by the same amount.
It cannot be overstated or overemphasized: one cannot simply add up gold + demand deposits + time deposits to obtain the “money” in the banking system. It may be helpful to think of the analogy of trying to add up 1/2 + 3/8 + 5/19 = 9/29.
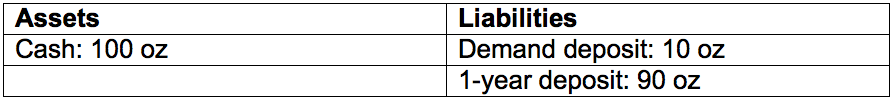
OK, now, let’s look at borrowing short to lend long, otherwise known as duration mismatch. Let’s say the depositor specified 10 oz on demand, and 90 oz to be withdrawn in 1 year. This balance sheet is:
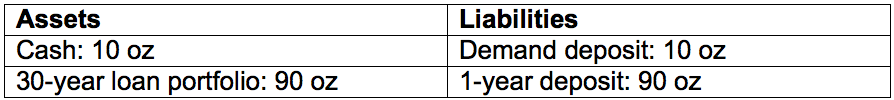
Up until this point, the bank is OK. Its assets are of zero duration (i.e. gold in the vault) and it has a portion of its liabilities of zero duration (i.e. demand deposit) and a portion that it must be able to pay in one year. The gold in the vault is obviously good for this (not counting that the gold is earning no interest, and the deposit must be paid back with interest). But let’s say the bank lends 90 ounces for 30 years, perhaps for a mortgage.
This bank has, at the very least, a liquidity problem. In one year, the depositor will come back asking for his 100 oz of gold. The bank has only 10. The other 90 oz worth is locked into a long-term mortgage. The bank will have to do something in order to be able to pay the depositor and avoid bankruptcy. I’ll discuss that further, below.
As the bank discovers when the depositor wants his money back, a mortgage is not money that can be used to pay expenses. The depositor wants gold, not a paper instrument.
So what does the bank do when presented by the demand from the depositor for his money? They find another depositor. This is one form of “rolling” the deposit from the first depositor to the second. Most of the time it works. In this example, the bank must plan to do this 30 times.
Usually, the banks can borrow fresh money to pay off loans that are due. There are many forms of rolling expiring loans, not necessarily involving depositors, but all have the same problem.
It is a confidence game, and of course it doesn’t work when there is stress in the system. Let people start to question the bank’s solvency, or solvency in general in the banking system, and depositors on net will withdraw their gold from the system and refuse to re-deposit it until they feel more comfortable. Duration mismatch will necessarily cause depositors to lose confidence sooner or later.
The issue is not merely that the bank is taking a risk. Duration mismatch is much like a check kiting scheme. It is a major factor contributing to the business cycle, which is actually a credit cycle of credit-expansion boom followed by credit-contraction bust.
So what have we concluded? First, fractional reserve lending is about lending less than the deposits a bank takes. The only party capable of creating money out of thin air is a central bank.
Second, fractional reserves do not necessarily cause any problems to the bank. If a depositor wants to liquidate a time deposit before maturity, the bank will seek the best bid in the market—and hand the loss off to the depositor.
Third, one cannot simply add up the various kinds and durations of banking deposits to come up with a simple (scalar) total. Gold is money. A demand deposit can be redeemed for money at any time. A time deposit that matures next year or in 2043 is not money; it may be convertible to money at an unknown loss. Thus banks can expand credit in the system but not money.
Finally, borrowing short to lend long, aka duration mismatch, inevitably implodes. This is not a matter of odds or probability. Like a geological fault line, one can try to assess probability of a destructive event in any given year, but sooner or later catastrophe is certain.
Duration mismatch is a very serious problem. Without identifying it separately, many people blame fractional reserve banking. This error would have massive repercussions, as banks play a vital role in a healthy economy.